Probability is one of those concepts that can be hard to wrap your head around. And even if you do, it doesn’t mean you understand how to use it in your everyday life. That’s where Roulette mathematics comes in. Roulette mathematics is a set of formulas and strategies used in Roulette games to improve your odds of winning. In this blog post, we will explore the basics of Roulette mathematics and provide tips on how to use them to improve your game.
Probability

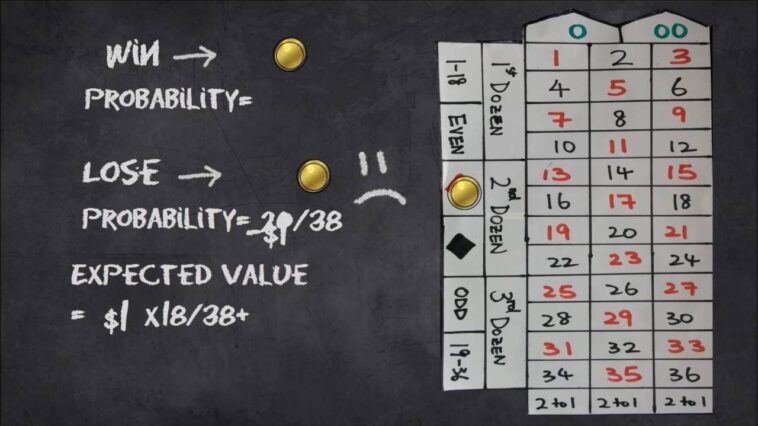
Probabilities are a fundamental part of Roulette betting. To understand probabilities, it’s important to first understand the basic principles of probability. Probability is a measure of how likely something is to happen. In other words, it tells you how likely each possible outcome is. For example, let’s say you have a deck of 52 cards. You can put them in any order you want and then randomly draw one card. What is the probability that the drawn card is an Ace? So the probability of getting an Ace is 1 out of 52 (or 2%).
Now let’s say you play online slot games such as Roulette and bet on the numbers 1-26. What is the probability of getting any number between 1 and 26? There are 36 possible numbers in that range, so the probability of getting any number between 1 and 26 is 36 out of 100 (or 36%). Let’s say you want to bet $1 on each number. The total amount you would win if you were correct is $36.
But by betting more money, your odds increase. That’s why it is important to understand probabilities when betting on Roulette games.
Odds

What are the odds of any given event occurring? How can you use mathematics to improve your chances of winning? In this article, we will explore these questions and more.
First, it is important to know the different types of odds. There are mathematical odds, betting odds, and house edge odds. Mathematical odds reflect how likely it is for an event to occur. Betting odds reflect how much you have to bet in order to win a given amount of money back when playing a game such as Roulette. The house edge reflects how much the casino profits from each bet placed.
Next, it is necessary to understand probability theory in order to make informed Roulette bets. Probability theory helps us understand why some outcomes are more likely than others and allows us to make better betting decisions. For example, if we know that the chance of receiving two black numbers in a row on a Roulette table is 6 in 100 (6%), then our best bet would be on black number 18 (18% chance). If we only cared about winning money rather than losing minimization, our best bet would be on any one of the other black numbers at this point (e.g., 16%, 15%, etc.).
However, by understanding probability theory and knowing that there is a greater chance of getting two black numbers in a row (due to the higher mathematical likelihood), we can make smarter Roulette bets based on our knowledge of probabilities rather than just blindly guessing which number to bet on.
Strategies

There are a variety of strategies that can be employed while playing Roulette, depending on the player’s preferences and personal experience. These include bet sizing, betting patterns, and strategy tables. While there is no guaranteed way to win at Roulette, following some basic tips and playing smart can increase the odds of coming out on top.
One important factor to consider when betting on Roulette is bet sizing. Players should avoid placing all their money on one number or color and instead spread their bets around to give themselves more opportunities for winning. Betting patterns can also help players improve their chances of winning by aiming for specific numbers or colors over time. Finally, strategy tables can offer guidance on how best to play a particular game or session – this information can be particularly helpful for new players who may not know which bets to make or how often to switch between them.
Systems Thinking in Roulette

Systems thinking is a valuable tool for understanding and improving Roulette strategies. By applying systems thinking principles, you can identify the patterns and relationships that underlie the behavior of Roulette systems. This information can help you make better bets, choose better odds, and improve your overall strategy.
The basic concepts of systems thinking can be applied to any system – from a single molecule to an entire economy – in order to gain an understanding of how that system works. In Roulette, this understanding allows you to make informed decisions about how to play the game.
One of the most important components of a successful Roulette strategy is understanding how probability works. Probability is the probability of an event happening given that certain conditions are current. For example, if you roll a 6 on a six-sided die, this means there is a 6% chance that you will get this result (assuming that all other conditions remain unchanged).
Roulette probabilities can be analyzed using various mathematical models such as binomial distribution or Poisson distribution. These models allow you to determine what are called “odds” – which represent the chances of getting a specific outcome in a particular number of rolls.
Relatedly, Roulette strategies also involve calculating “payoffs.” A payoff is simply what you win or lose when playing a particular strategy – it’s basically your share of the prize pool if you win, and your losses if you lose. Payoffs can be expressed as percentages or dollar values.
Conclusion
In this final article in our series on Roulette mathematics, we will be discussing probability, odds, and strategies. Probability is the measure of how likely something is to happen. Odds represent the relative chances of two or more outcomes occurring. Strategies are ways to improve your chances of winning at Roulette by understanding those odds and making wise decisions. Last but not least, systems are models that help you make predictions about how the game will play out based on past results. By applying these concepts to Roulette, you can improve your chance of winning big!